VI) Development and Application of Relativistic Methods
1. Development of Relativistic DFT and Relativistic Wave-function Methods
(Project leader: Michael Filatov, Dieter Cremer)
Relativistic corrections are important for any atom with an atomic number larger than 18 (although strong relativistic effects are experienced only above Z = 36) and therefore more than 80% of the elements of the periodic table require a relativistic quantum chemical description to obtain reliable results. The inclusion of relativistic corrections into a quantum chemical calculation increases the computational efforts significantly. Therefore, it was the major purpose of our work to include relativistic effects in such a way that large-scale, all-electron calculations for relatively large molecules are still possible. The inclusion of relativistic effects at the all-electron level is needed for the relativistic calculation of NMR chemical shifts, nuclear spin-spin coupling constants, and other magnetic properties, which depend on the inner (core) electrons.
In the last four years, we developed a hierarchy of methods (Figure C) with the goal to approximate the solution of the Dirac equation as accurately as possible. We demonstrated that the regular approximation to the relativistic Hamiltonian provides the best starting point to lead from zeroth order (ZORA) via an infinite order approach (IORA) directly to the unnormalized and normalized elimination of the small component (NESC) and there again by increasing (within NESC) the orders of the perturbational description to the exact solutions of the Dirac equation. At present, we have reached by developing NESC-SORA (second order regular approximation) a level of accuracy, which easily beats rival methods such as the Douglas-Kroll-Hess method in fifth order at much lower cost.
This development was only possible by solving three major problems typical of the regular approximation: a) The gauge-dependence problem. b) The difficulties of applying the regular approximation to correlation-corrected wave-function methods. c) The positioning of the regular approximation within a hierarchy of relativistic methods. - Problem a) was solved by inventing methods such as IORAmm (IORA with modified metric), scaled IORA and finally ZORA-GI and IORA-GI (GI: gauge independent) that suppress gauge-dependence errors stronger and stronger. For problem b) a matrix formulation of the key equations was found that opened a route to correlation-corrected wave-function methods of increasing sophistication. Recently, problem c) was solved by showing for the first time the close relationship between the regular approximation and NESC equations and by this the Dirac equations.
In Figure C, the relativistic methods developed in the last four years are ordered in increasing sophistication thus approaching the Dirac description. Those shown on a blue background contain a correction to suppress the gauge-dependence problem. In the horizontal direction DFT and WFT (wave-function theory) methods are grouped which include correlation corrections. Relativistic versions of these methods are available as indicated by a cross (X). For the majority of the methods, analytical first derivatives were developed to calculate routinely geometries and first order properties such as dipole moments, multipole moments, etc. (red cross). In some cases, we worked out analytical second derivatives for the routine calculation of vibrational frequencies, infrared intensities, polarizabilities, etc. (purple cross). Second order properties such as magnetic shieldings, NMR spin-spin coupling constants or ESR hyperfine coupling constants require also analytical second derivatives, but in addition also a special program coding. This could be installed already for some relativistic correlation-corrected methods (blue cross).
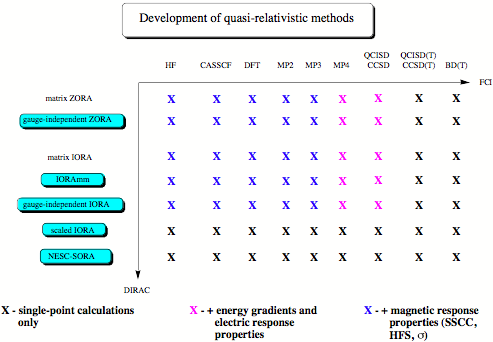
Figure C. Overview of relativistic methods developed in the last four years. The vertical axis orders the relativistic approximations according to increasing accuracy. The horizontal axis orders the wavefunction methods, which have been relativistically corrected according to increasing accuracy relative to the exact full CI result (FCI). For completeness DFT has been included in this order. Explanations: 1) ZORA (zeroth order regular approximation); 2) NESC-EP (normalized elemination of the small component with an effective potential); 3) IORA (infinite order regular approximation); 4) IORAmm (IORA with metric matrix); 5) HF (Hartree-Fock); 6) MPn (n-th order perturbation theory with the Møller-Plesset perturbation operator); 7) CCSD (Coupled-Cluster theory with all single (S) and double (D) excitations); 8) CCSD(T) (CCSD with a perturbational inclusion of the triple (T) excitations); 9) BD(T) (Coupled Cluster theory with Brückner orbitals, all D excitaions, and a pertubational inclusion of the T excitations: 10) CASSCF (complete active space self consistent field); 11) FCI (full configuration interaction). Black crosses: only energy calculations possible. Red crosses: analytical first derivatives available for geometries and other first order properties. Purple crosses: also analytical second derivatives available for vibrational frequencies and other second order properties (apart from magnetic properties). Blue crosses: also derivatives for magnetic properties.
Figure D. Various application projects carried out with the new relativistic methods.
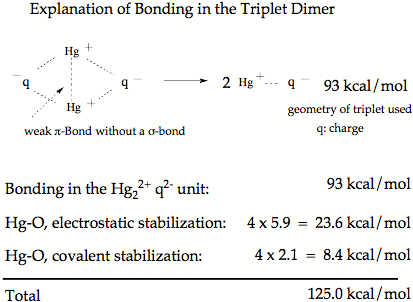
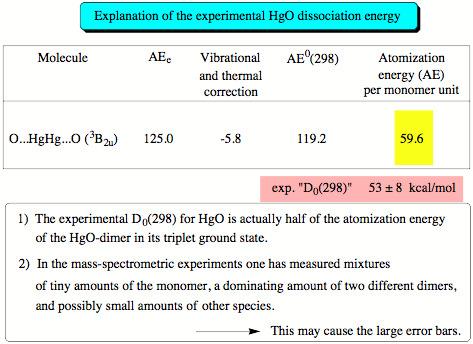
2. Investigation Quantum Dot Materials with Relativistic Methods
(Michael Filatov, Dieter Cremer)
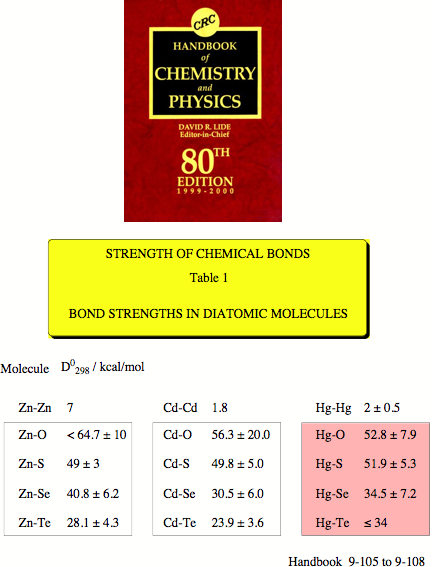
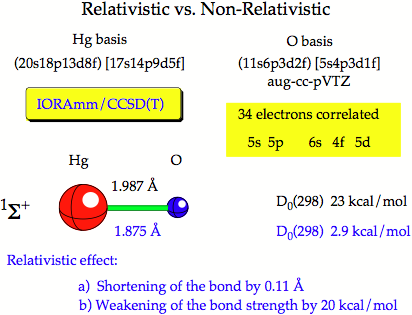
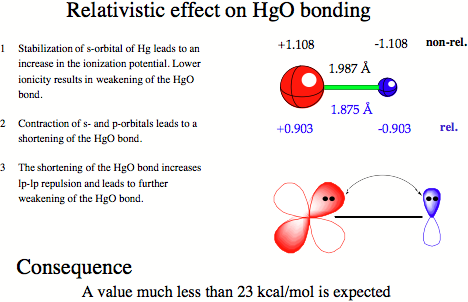
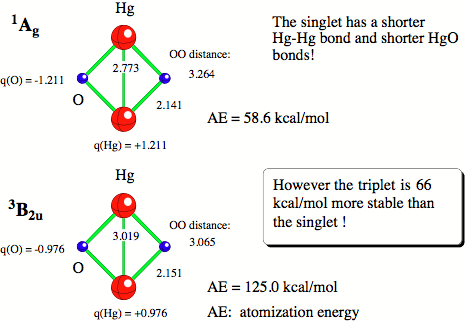
Mercury chalcogenides HgE (E = O, S, Se, etc.) are described in the literature to possess rather stable bonds with bond dissociation energies between 53 and 30 kcal/mol, which is actually difficult to understand in view of the closed shell electron configuration of the Hg atom in its ground state (…4f145d106s2). Based on relativistically corrected many body perturbation theory and coupled cluster theory (IORAmm/MP4, Feenberg-scaled IORAmm/MP4, IORAmm/CCSD(T)) in connection with IORAmm/B3LYP theory and a [17s14p9d5f]/aug-cc-pVTZ basis set, we demonstrate that the covalent HgE bond is rather weak (2 - 7 kcal/mol), the ground state of HgE is a triplet rather than a singlet, and that the experimental bond dissociation energies have been obtained for the dimers (or mixtures of monomers, dimers, and even trimers) Hg2E2 rather than true monomers. The dimers possess association energies of more than 100 kcal/mol due to electrostatic forces between the monomer units. The covalent bond between Hg and E is in so far peculiar as it requires a charge transfer from Hg to E (depending on the electronegativity of E) for the creation of a single bond, which is supported by electrostatic forces. However, sigma-bonding between Hg and E is reduced by strong lone pair - lone pair repulsion to a couple of kcal/mol. Since a triplet configuration possesses somewhat lower destabilizing lone pair energies, the triplet state is more stable. In the dimer, there is a Hg-Hg pi-bond of bond order 0.66 without any sigma-support. Weak covalent Hg,O interactions are supported by electrostatic bonding. - The results for the mercury chalcogenides suggests that all experimental dissociation energies for group 12 chalcogenides have to be revised because of erroneous measurements.